The range of mathematics and technical competencies that forms my area of interest is often beyond my scope. In such cases, I collaborate with artists and academics with similar interests but different expertise.
After my last sabbatical trip to examine the Constance Howard Textiles Collection at Goldsmiths College, I formed a research group that focuses on educational applications of the mathematics in textiles.
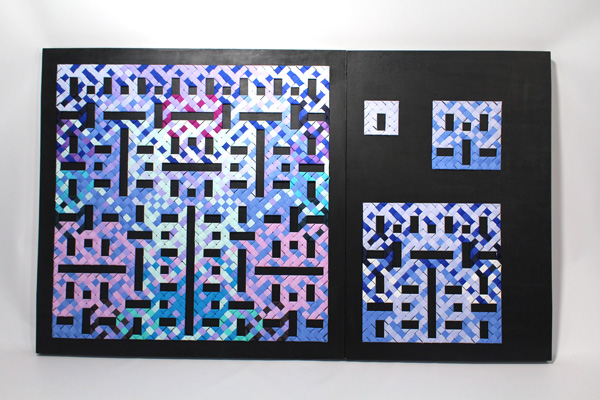
In particular, recent art-generating work has involved exploring what forms can be created using paper-strip weaving, applying a variety of traditional techniques from around the world that were exemplified in the collection. More details can be found in our dedicated website.
The image shows a series of paper-strip woven pieces that explore colour-and-weave effects.

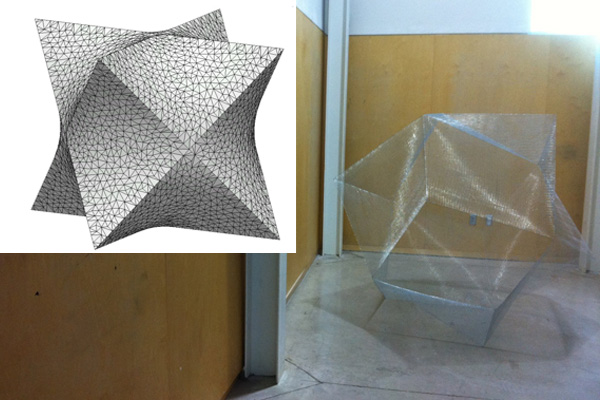
Nathan Wilson was an MFA student in sculpture at NSCAD. With his help, I explored forms derived by combining polyhedra with saddle surfaces. He assembled galvanised metal mesh squares into configurations derived from the placement of faces, edges and vertices in the regular and semi-regular polyhedra.
The image shows the case of the (anti-)cube.
The Animated Riffs on 1870s Galloping Horse Footage, produced by Carnegie Mellon University students so intrigued me and Sibylle Herzer (artist) that we decided to make our own version of the project.
Instead of producing a series of animations or a galloping horse, we are creatibng works in as many media aswe can, based on the photograph of a starfish in a tide pool, taken on the Eastern Shore of Nova Scotia.
The image shows a rendering using the Colourwave schema.

Twining and braiding rope is a spacial experience that demonstrates potential for mathematical learning. With Susan Gerofsky (mathematics educator) and James Forren (architect), I am investigating the cognitive benefits of participating in the manual/group making of braided and twined structures, including both ropes and architectural forms. More information can be found in our dedicated website.
The image shows a still from a braiding machine that mimics some traditional dances such as square-dancing.

During the course of my last sabbatical trip to London, UK, I came across a row of houses that sported a decorative feature that intrigued me for some of its aesthetic and mathematical properties.
As a result of my investigations into the tiles, some mathematical problems are being raised, which Dr Danielle Cox and Dr Karyn McLelland (mathematicians) are helping me solve. The exploration combines:
The image shows one of the façades, some of the tools used in the investigation, and two of the pieces that were produced so far: an indigo resist-dyed print and an inkle-woven strip.

D=forms were developed by Tony Wills, and are defined as 3-dimensional forms created by connecting the edges of two flat, non-stretching and usually convex surfaces of equal perimeter.
In 2008, John Sharp, Roger Tobie and I explored the potential of using Cassini-ovals to create D-forms. This culminated in a workshop for building a Calder-style mobile based on the shapes produced.
The image shows a series of D-forms made by unstitching and re-stitching soft-ball patches, starting at different points.

In the early-to-mid 2000s, the Su Doku became all the rage. Though it is strictly speaking more logical reasoning than mathematics, it is interesting to consider the reasoning in which a player engages. If colours are substituted for the numbers, the experience of solving the puzzle becomes different again as anytime a result is found by elimination, this is made easier in that coloured pencils or markers are simply lain aside during the reasoning cycle.
With Dr Mary Crowley (quilter and math educator) I designed and created a Su Doku quilt based on this idea. The image shows the puzzle and its solition, together with a sampling of cloth-covered buttons that stand in for the markers.

The premise of traditional Origami is that nothing is cut and nothing is measured. Relationships between folds are therefore based on relationships in the original paper's shape and dimensions. After playing with this idea using circular paper, I developed a technique to make any deltahedron, i.e. any polyhedron made of only equilateral triangles.
With Dr Simon Morgan (mathematician), I then devised a large-scale version of these shapes, particularly the original one, the “endo-pentakis-icosi-dodecahedron”. This culminated in a participatory Barn-Raising event which involved the erection of the 80-sided polyhedron composed of colourful equilateral triangles, from over 100 pieces.
The event initially took place in two locations: in Texas in a middle school and in Kansas at an art and mathematics conference. The modular elements have since been used in conferences and educational contexts from Kindergarten through Graduate School, in multiple countries (More details can be found in the dedicated website.).
The image shows “Geraldine”, in situ in the courtyard of the Middle School.

as part of my work on the mathematics in weaving, I have been investigating a technique, used in the South Pacific Islands, that involved creating openings in a woven mat, without cutting, by reversing directions with selected woven elements.
Based on this idea, and with the assistance of Dr Wendy Landry (weaver) and Dr Simon Morgan (mathematician), I was able to realise a large-scale, multi-iteration version of one of my variations on the Hilbert Curve.
The image shows the first four iterations of the Hilbert Curve, re-interpreted as a square region with holes, made from grosgrain ribbon interwoven on a polyropylene matrix. The colouring shows the paths that are isometrically equivalent.
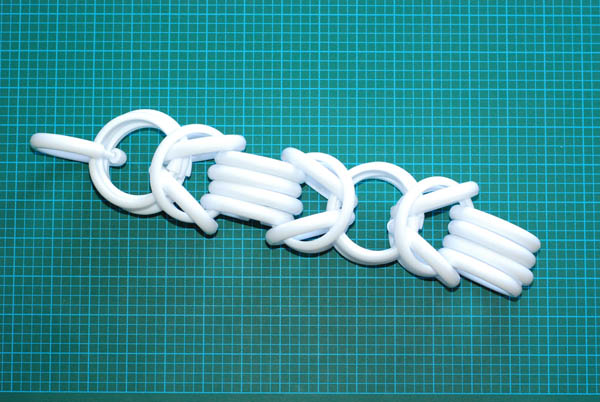
Chains come in a multitude of varieties, not all of which are based on the single loop link.
Dr Tara Taylor is a mathematician. Together, we defined "chains" mathematically as “a series of inter-connected, rigid elements called links which, together, constitute a linear, flexible (non-rigid) object that can be used to connect, wind, hang or wrap”. From there, we investigated the mathematical properties of the examples we could find.
Chains constitued an interesting field of mathematical enquiry, especially from a topological perspective. This culminated in a workshop at an art and mathematics conference.